평면그래프,planar_graph

Difference between r1.6 and the current

@@ -1,7 +1,16 @@
'''평면그래프, planar graph'''
대충, 평면 위에(ex. 종이 위에) edge를 겹치지 않고 그릴 수 있는 graph?

성질
[[종수,genus]]가 0
MKL
[[평면,plane]]
[[embedding]] KmsE:embedding WtEn:embedding https://ncatlab.org/nlab/show/embedding
CMP
[[polyhedral_graph]] { polyhedral graph (번역참고 KmsE:polyhedr ) (MKL WtEn:Herschel_graph ) Twin: MathWorld:PolyhedralGraph WpEn:Polyhedral_graph WpJa:多面体グラフ "polyhedral graph" Ggl:"polyhedral graph" }

= quasi-planar graph =
[[quasi-planar_graph]]
@@ -9,14 +18,24 @@

= tmp bmks =
https://math.stackexchange.com/questions/3073581/formal-definition-of-planar-graph
http://discrete.openmathbooks.org/dmoi3/sec_planar.html

= tmp =
// from wpen
[[평면,plane]]에 embed될 수 있는(graph_embedding - [[WpEn:Graph_embedding]]) 그런 그래프가 '''평면그래프'''.
i.e. 1. [[edge]]s들이 endpoint에서만 만나며, 중간에 겹치지(intersection) 않는, 그런 방식으로 평면에 그릴 수 있다.
i.e. 2. 어떤 edges들도 서로 교차하지(cross) 않는 방법으로 평면에 그릴 수 있다.
...
[[입체사영,stereographic_projection]] ....TBW LATER
----
// from 수학백과
QQQ "변들이 서로 공유하는 끝점에서만 만나도록" 뜻이??
QQQ "변들이 서로 공유하는 끝점에서만 만나도록" 정확한 뜻이??
''[[Date(2022-01-27T09:45:51)]] 이것은 '개념'과 '그림으로 그리는 것'이 서로 다름을 이해하면 바로 파악 가능, 좀 모호한 표현 같음.''
4색정리/4색문제 ,four_color_theorem { 현재 =,four_color 에 작성중 } 의 그 그래프.
언급:
* 오일러의 공식 $v(G)-e(G)+f(G)=2$
* 쿠라토프스키의 정리 Kuratowski { [[세분,subdivision]] } (평면그래프의 필요충분조건과 연관)
* 쿠라토프스키의 정리 Kuratowski { [[세분,subdivision]] { 세분 via KmsE:subdivision https://ncatlab.org/nlab/show/subdivision Ggl:subdivision } } (평면그래프의 필요충분조건과 연관)
* 플라톤 입체(Platonic solid) { 각 면의 모서리들의 개수가 모두 같고 각 꼭짓점에 들어가는 모서리의 개수가 모두 같은 볼록다면체 }
이건 국내에서 흔히 [[정다면체]]라고 하는 바로 그것. curr at [[기하학,geometry]] 앞부분. later at [[다면체,polyhedron]].
----
@@ -26,15 +45,23 @@
https://ncatlab.org/nlab/show/Euler%27s+formula+for+planar+graphs

Google:평면그래프+오일러
----
Cmp: [[평면곡선,plane_curve]]
----
Twins:
[[https://terms.naver.com/entry.naver?docId=3405389&cid=47324&categoryId=47324 수학백과: 평면그래프]]
https://mathworld.wolfram.com/PlanarGraph.html
https://proofwiki.org/wiki/Definition:Planar_Graph
[[WpKo:평면_그래프]]
[[WpEn:Planar_graph]]
https://ncatlab.org/nlab/show/planar+graph
[[Libre:평면그래프]]
https://encyclopediaofmath.org/wiki/Graph,_planar

이름에서 관련: [[평면,plane]]
이 그래프의 성질을 명사화하면 그래프평면성 graph_planarity 정도? planarity의 뜻: https://en.wiktionary.org/wiki/planarity

Up: [[그래프,graph]]


평면그래프, planar graph

대충, 평면 위에(ex. 종이 위에) edge를 겹치지 않고 그릴 수 있는 graph?

성질
종수,genus가 0


CMP
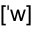polyhedral_graph { polyhedral graph (번역참고 KmsE:polyhedr ) (MKL WtEn:Herschel_graph ) Twin: MathWorld:PolyhedralGraph WpEn:Polyhedral_graph WpJa:多面体グラフ "polyhedral graph" Ggl:polyhedral graph }

[edit]

quasi-planar graph

[edit]

tmp bmks

[edit]

tmp

// from wpen
평면,plane에 embed될 수 있는(graph_embedding - WpEn:Graph_embedding) 그런 그래프가 평면그래프.
i.e. 1. edges들이 endpoint에서만 만나며, 중간에 겹치지(intersection) 않는, 그런 방식으로 평면에 그릴 수 있다.
i.e. 2. 어떤 edges들도 서로 교차하지(cross) 않는 방법으로 평면에 그릴 수 있다.
...
입체사영,stereographic_projection ....TBW LATER
// from 수학백과
QQQ "변들이 서로 공유하는 끝점에서만 만나도록" 정확한 뜻이??
2022-01-27 이것은 '개념'과 '그림으로 그리는 것'이 서로 다름을 이해하면 바로 파악 가능, 좀 모호한 표현 같음.

4색정리/4색문제 ,four_color_theorem { 현재 =,four_color 에 작성중 } 의 그 그래프.
언급:
///from https://www.youtube.com/watch?v=kxg8u1UU6LI
7m. 평면그래프는 오일러의 정리(see 그래프색칠,graph_coloring - 불변량 섹션)에서 $v-e+f=2$ (평면그래프의 모든 vertex - edge + face = 2)





이름에서 관련: 평면,plane
이 그래프의 성질을 명사화하면 그래프평면성 graph_planarity 정도? planarity의 뜻: https://en.wiktionary.org/wiki/planarity